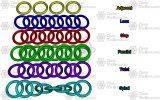
This thread is for the general discussion of the Weave Leanelated. Please add to the discussion here.
I am having a really hard time trying to reduce this to tesselation and cells.
If I am reading this correctly, this is a 4:4 weave, looking very much like its 6:3/3:6 sibling; namely each cell is two large rings, intersected with 2 small rings - and while I say they share a total of 8 small rings, you’ll probably contest me and show how to distribute them to connect to the neighbours.
If I am reading this correctly, this is a 4:4 weave, looking very much like its 6:3/3:6 sibling; namely each cell is two large rings, intersected with 2 small rings - and while I say they share a total of 8 small rings, you’ll probably contest me and show how to distribute them to connect to the neighbours.
Hahahahahahaha, you know it
well, yes and no
6 small rings.
There are a few different ways to describe this weave structurally. There are 4 different grain transformations possible with this weave:
-
Leanelated - Lean grain
- Crenelated-3 - Step grain
- Borealis - Parallel grain
- Twistelated - Twist grain (currently undescribed because the correct ARs are still being worked out)
While the above are the base of the weave, to make weave cells, you need at least to of them so you can show the way the cells are connected. I'm going to leave out Twistelated because that has an extra connection that the others do not (which I'm pretty sure changes the base to European 3 in 1). I'm also only going to show building in one direction, it can be done in the opposite direction as well.
These are one of the possible weave cells for the three weaves
From this, we can also figure out the base chains necessary for each of the weaves:
- Leanelated - European 4 in 1 (Dilated Band form)
- Crenelated - Gridlock Sheet (Dilated Band form)
- Borealis - Celtic
Please let me know if you would like further explanation
There are several things that I contest here.
For me, crenelated is a 3:6 weave at the base, and not a 4:4, and borealis is a 3:6 weave at the base. Using subcells, crenelated is 3:6, disregarding subcells as a concept. It’s 6:3.
There’s a huge difference between leanelated and the other two. I am sorry, as I miscounted - it’s of course 10 small rings according to my method, sharing all but 2 (that comes down to 6 rings in total per cell) - but viewing the larger ring as a triangle - leanelated repeats as a pair of triangles forming a parallelogram <> - no smaller shape can be added without changing the pattern, and parallelograms are just distorted squares, making the weave extend with an average of 90 degree rotation
Crenelated can be approximated as 6 triangles, or as with borealis as a hexagon for the smallest repeating (sub)cell, as such, it extends with 60 degrees rotation.
borealis, for me really shows why rings are shared between cells. Each sandwich pair shares 3 sandwiched rings with 3 other sandwich pairs. I know you always try to convince me otherwise, but this cements my view.
For me, crenelated is a 3:6 weave at the base, and not a 4:4, and borealis is a 3:6 weave at the base. Using subcells, crenelated is 3:6, disregarding subcells as a concept. It’s 6:3.
There’s a huge difference between leanelated and the other two. I am sorry, as I miscounted - it’s of course 10 small rings according to my method, sharing all but 2 (that comes down to 6 rings in total per cell) - but viewing the larger ring as a triangle - leanelated repeats as a pair of triangles forming a parallelogram <> - no smaller shape can be added without changing the pattern, and parallelograms are just distorted squares, making the weave extend with an average of 90 degree rotation
Crenelated can be approximated as 6 triangles, or as with borealis as a hexagon for the smallest repeating (sub)cell, as such, it extends with 60 degrees rotation.
borealis, for me really shows why rings are shared between cells. Each sandwich pair shares 3 sandwiched rings with 3 other sandwich pairs. I know you always try to convince me otherwise, but this cements my view.
Forgetting leanelated for a minute, as shown, what would you call the red and green examples in the last render?
Something akin to non-cell incomplete sections of Crenelated and Borealis.
I think you're equating weave cells with segments or units (both of which I haven't posted how they work in CCT). Any weave will need a minimum number of weave cells translated on the appropriate axis before the weave form is recognizable.
are these also incomplete sections?
are these also incomplete sections?
Ah, you meant the very last.
No, those contain all subsegments that will produce the weave.
No, those contain all subsegments that will produce the weave.
Just noticed that you said this:
)
Are you referring to something like this for Crenelated and Borealis?
Because I have some news for you:
That's the beauty and the horror of chainmaille, there are multiple ways to describe a weave, just as there are multiple ways to assemble weaves. I describe weaves based on CCT (which I will continue to write articles about to try and explain it further).
In CCT, there is NO rotation between weave cells, ONLY translations (except in spiral grains, but grains are an entirely different aspect that will be discussed in yet another article
)Are you referring to something like this for Crenelated and Borealis?
Because I have some news for you:
For you (and everyone else), any weave can be whatever you want
That's the beauty and the horror of chainmaille, there are multiple ways to describe a weave, just as there are multiple ways to assemble weaves. I describe weaves based on CCT (which I will continue to write articles about to try and explain it further).In CCT, the ONLY difference between these three weaves are the grains that the large rings form:
-
Leanelated is a Lean grain \\\\\\\\ - Crenelated is Step grain _-_-_-
- Borealis is Parallel grain -=-=-=
Then CCT is noway near enough, I'm sorry. Leanelated introduces an element that really changes the topology. The topology for crenelated can be reduced to the single ring with 6 small rings working as a triangle. The topology for borealis requires what you posted. As you see for both of them, you can rotate them 120 degrees and you'll be where you started. With Leanelated, you won't, you need a 180 degree rotation (and a flip). If you rotate borealis 180 degrees, you do not have the same edge towards you, you need to rotate 60 degrees in either direction and a flip won't change that.Just noticed that you said this:
In CCT, there is NO rotation between weave cells, ONLY translations (except in spiral grains, but grains are an entirely different aspect that will be discussed in yet another article
Are you referring to something like this for Crenelated and Borealis?
View attachment 8711
Because I have some news for you:
View attachment 8712
For you (and everyone else), any weave can be whatever you want
In CCT, the ONLY difference between these three weaves are the grains that the large rings form:
- View attachment 8713 Leanelated is a Lean grain \\\\\\\\
- Crenelated is Step grain _-_-_-
- Borealis is Parallel grain -=-=-=
Then CCT is noway near enough for me, I'm sorry.
Fixed that for you.
As I've said multiple times CCT is a different way of looking at things and it may not be everyone's cup of tea. You are comparing apples and oranges.
Using CCT, by simply changing where the base cells are connected I can show how you can go from a Leanelated unit to a Crenelated unit and from a Crenelated unit to a Borealis unit by modifying it with the addition of the extra adjacent layer and an increase in AR of the smaller rings to allow for that extra layer. This is just 2 out of the 3 cells needed to create units of each weave.
- If the light blue ring in leanelated under the dark blue ring, it would be Crenelated.
- If I put the orange ring over the red ring in Crenelated, it would be Leanelated.
- If I put another red and orange under the first and third rings AND parallel with the second and fourth, (or over the second and fourth and parallel with the first and third) in Crenelated, it would be Borealis.
- If I took away the bottom large rings OR the top large rings in Borealis, it would be Crenelated.
and what? You're comparing a segment of Leanelated sheet to a unit of Crenelated and a unit of Borealis. With Crenelated and Borealis sheets, the segments are the same as the units, with leanelated they are not. Please note, I am talking based on CCT definitions. If I make a unit (as per CCT, because that's how I do things) what you state above is incorrect.
Top Row - Left side is a segment of Leanelated sheet, right side is a unit of Leanelated
Bottom Row - Left side is a segment of Leanelated sheet rotated 120 degrees on a central axis, right side is a unit of Leanelated rotated 120 degrees on a central axis:
